지난 글에서 유도한 응력의 변환방정식은 아래와 같습니다.
$\sigma_{x'}=\frac{\sigma_{x}+\sigma_{y}}{2}+\frac{\sigma_{x}-\sigma_{y}}{2}\cos 2\theta+\tau_{xy}\sin 2\theta$
$\tau_{x'y'}=-\frac{\sigma_{x}-\sigma_{y}}{2}\sin 2\theta+\tau_{xy} \cos 2\theta$
변수는 $\theta$ 입니다. $\theta$로 미분한 함수가 0이 되는 $\theta$ 에서 극값이 발생합니다.
첫번째 식을 $\theta$ 로 미분하면 아래와 같습니다.
$\frac{d\sigma_{x'}}{d \theta}=-(\sigma_{x}-\sigma_{y})\sin 2\theta+2\tau_{xy}\cos 2\theta=0$
이항하면 아래와 같습니다.
$2\tau_{xy}\cos 2\theta=(\sigma_{x}-\sigma_{y})\sin 2\theta$
아래와 같이 변형합니다.
$\frac{\sin 2\theta}{\cos 2\theta}=\frac{\tau_{xy}}{\frac{(\sigma_{x}-\sigma_{y})}{2}}$
좌변은 탄젠트입니다.
$\tan 2\theta=\frac{\tau_{xy}}{\frac{(\sigma_{x}-\sigma_{y})}{2}}$
위 등식을 만족하는 $\theta$ 를 구할 필요는 없습니다. 아래그림의 $\theta_{1}$과 $\theta_{2}$가 위 등식이 만족한다는 것을 알면 됩니다.
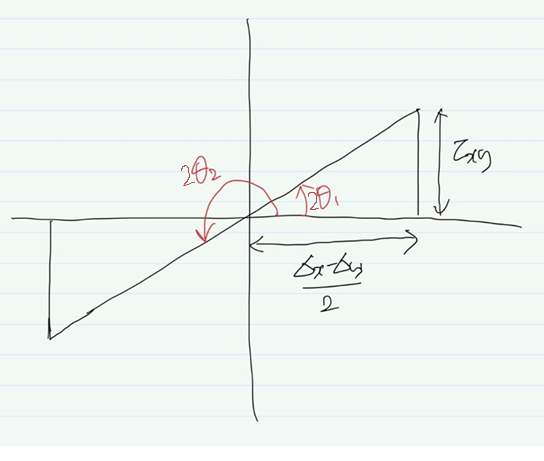
또한 $\theta_{1}$과 $\theta_{2}$는 90도 차이임을 알 수 있습니다.
$\theta=\theta_{1}$일 때 수직응력의 변환방정식은 아래와 같이 변형됩니다. 위 그림에서 $\sin 2\theta_{1}$과 $\cos 2\theta_{1}$을 구하여 넣어주었습니다.
$\sigma_{x'}=\frac{\sigma_{x}+\sigma_{y}}{2}+\frac{\sigma_{x}-\sigma_{y}}{2}
\frac{\frac{\sigma_{x}-\sigma_{y}}{2}}{\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}}
+\tau_{xy}
\frac{\tau_{xy}}{\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}}$
아래와 같이 계산합니다.
$\sigma_{x'}=\frac{\sigma_{x}+\sigma_{y}}{2}
+
\frac{ \left (\frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2} }{\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}}$
아래와 같이 변형합니다.
$\sigma_{x'}=\frac{\sigma_{x}+\sigma_{y}}{2}
+
\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}$
$\theta=\theta_{2}$일 때도 같은 방법으로 구하면 아래와 같습니다.
$\sigma_{x'}=\frac{\sigma_{x}+\sigma_{y}}{2}
-
\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}$
두 값을 주응력이라고 합니다. 값이 큰 응력을 $\sigma_{1}$, 값이 작은 응력을 $\sigma_{2}$ 라고 놓습니다.
$\sigma_{1,2}=\frac{\sigma_{x}+\sigma_{y}}{2}
\pm
\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}$
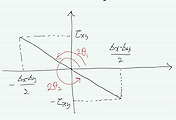
주응력 상태에서 전단응력은 어떤 값을 가질까요? $\theta_{1}$과 $\theta_{2}$에서 전단응력을 구하면 0이 나옵니다. 구하는 방법은 어렵지 않습니다. 전단응력변형공식에 위에서 구한 사인과 코사인 값을 대입해서 구하면 됩니다. $\theta_{1}$과 $\theta_{2}$는 90도 차이이므로 최대주응력상태에 있는 요소는 아래와 같이 나타낼 수 있습니다.
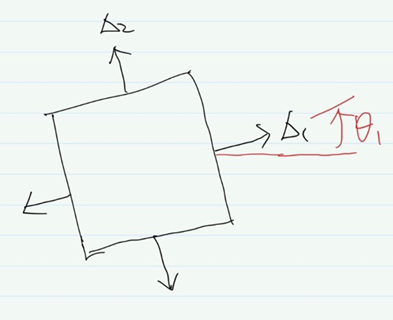
아래는 요약입니다.
1. 주응력은 수직응력 변환공식을 미분한 값이 0이 될 때 발생하며, 발생 각도와 주응력은 아래와 같다.
$\tan 2\theta=\frac{\tau_{xy}}{\frac{(\sigma_{x}-\sigma_{y})}{2}}$
$\sigma_{1,2}=\frac{\sigma_{x}+\sigma_{y}}{2}
\pm
\sqrt{\left ( \frac{\sigma_{x}-\sigma_{y}}{2} \right )^{2}+\tau_{xy}^{2}}$
2. 주응력 상태에서 전단응력은 0임
3. $\theta_{1}$과 $\theta_{2}$는 90도 차이이고, 주응력상태에서 전단응력은 0이므로 아래와 같이 나타낼 수 있다.

'재료역학 > 평면응력과 응력 변환' 카테고리의 다른 글
| [재료역학] 평면응력 (6) 모어원 유도 (0) | 2022.06.15 |
|---|---|
| [재료역학] 평면응력 (5) 최대전단응력 예제 1 (0) | 2022.06.15 |
| [재료역학] 평면응력 (4) 주응력 예제 1 (0) | 2022.06.14 |
| [재료역학] 평면응력 (3) 최대전단응력공식 유도 (0) | 2022.06.10 |
| [재료역학] 평면응력 (1) 응력의 변환방정식 유도 (0) | 2022.06.07 |

댓글