[기구학] 4절 링크 위치해석 (position analysis) (1) 공식 유도

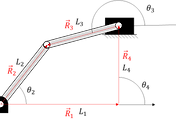
아래 그림과 같이 4절 링크가 있습니다. 주어진 값은 각 링크의 길이인 $L_{1}$,$L_{2}$,$L_{3}$,$L_{4}$와 $\theta_{2}$ 입니다. 이 값들을 이용하여 $\theta_{3}$, $\theta_{4}$ 를 구하는 것이 목적입니다.

 

 

벡터 방정식은 아래와 같습니다. 

 

$\vec{R}_{2}+\vec{R}_{3}=\vec{R}_{1}+\vec{R}_{4}$

 

아래와 같이 이항합시다. 

 

$\vec{R}_{2}+\vec{R}_{3}-\vec{R}_{1}-\vec{R}_{4}=0$

 

오일러 공식을 이용하여 복소평면의 극좌표로 변형하면 아래와같습니다. 

 

$L_{2}e^{j\theta_{2}}+L_{3}e^{j\theta_{3}}-L_{1}e^{j\theta_{1}}-L_{4}e^{j\theta_{4}}=0$

 

사인,코사인 형태로 나타내면 아래와 같습니다. 

 

$L_{2}\left ( \cos \theta_{2}+j\sin \theta_{2} \right ) +L_{3}\left ( \cos \theta_{3}+j\sin \theta_{3} \right ) -L_{1}\left ( \cos \theta_{1}+j\sin \theta_{1} \right ) -L_{4}\left ( \cos \theta_{4}+j\sin \theta_{4} \right )=0$

 

$\theta_{1}$은 0도이므로, 아래와 같이 변형됩니다. 

 

$L_{2}\left ( \cos \theta_{2}+j\sin \theta_{2} \right ) +L_{3}\left ( \cos \theta_{3}+j\sin \theta_{3} \right ) -L_{1} -L_{4}\left ( \cos \theta_{4}+j\sin \theta_{4} \right )=0$

 

실수부와 허수부를 따로 나타내면 아래와 같습니다. 

 

$L_{2}\cos\theta_{2}+L_{3}\cos\theta_{3}-L_{1}-L_{4}\cos\theta_{4}=0$

 

$L_{2}\sin\theta_{2}+L_{3}\sin\theta_{3}-L_{4}\sin\theta_{4}=0$

 

1) $\theta_{3}$ 구하기

$\theta_{4}$를 제거하기 위해 위 두 식을 각각 아래와 같이 이항해줍니다. 

 

$L_{4}\cos\theta_{4}=L_{2}\cos\theta_{2}+L_{3}\cos\theta_{3}-L_{1}$

 

$L_{4}\sin\theta_{4}=L_{2}\sin\theta_{2}+L_{3}\sin\theta_{3}$

 

위 두 식의 양변을 제곱하여 더해줍니다. 

 

$\begin{align} L_{4}^{2}\left ( \cos^{2}\theta_{4}+\sin^{2}\theta_{4} \right )=&L^{2}_{2}\cos^{2}\theta_{2}+L^{2}_{3}\cos^{3}\theta_{3}+L^{2}_{1}+2L_{2}L_{3}\cos\theta_{2}\cos\theta_{3}-2L_{1}L_{2}\cos\theta_{2}-2L_{1}L_{3}\cos\theta_{3}+ \\&L_{2}^{2}\sin^{2}\theta_{2}+L_{3}^{2}\sin^{2}\theta_{3} +2L_{2}L_{3}\sin\theta_{2}\sin\theta_{3}\end{align}$

 

$\cos^{2}\theta+\sin^{2}\theta=1$이므로 아래와 같이 변형됩니다. 

 

$L_{4}^{2}=L^{2}_{2}+L^{2}_{3}+L^{2}_{1}+2L_{2}L_{3}\cos\theta_{2}\cos\theta_{3}+2L_{2}L_{3}\sin\theta_{2}\sin\theta_{3}-2L_{1}L_{2}\cos\theta_{2}-2L_{1}L_{3}\cos\theta_{3}$

 

아래와 같이 이항합니다.

 

$L_{4}^{2}-L^{2}_{2}-L^{2}_{3}-L^{2}_{1}=2L_{2}L_{3}\cos\theta_{2}\cos\theta_{3}+2L_{2}L_{3}\sin\theta_{2}\sin\theta_{3}-2L_{1}L_{2}\cos\theta_{2}-2L_{1}L_{3}\cos\theta_{3}$

 

양변을 2로 나눕니다. 

 

$\frac{L_{4}^{2}-L^{2}_{2}-L^{2}_{3}-L^{2}_{1}}{2}=L_{2}L_{3}\cos\theta_{2}\cos\theta_{3}+L_{2}L_{3}\sin\theta_{2}\sin\theta_{3}-L_{1}L_{2}\cos\theta_{2}-L_{1}L_{3}\cos\theta_{3}$

 

양변을 $L_{2}L_{3}$로 나눕니다. 

 

$\frac{L_{4}^{2}-L^{2}_{2}-L^{2}_{3}-L^{2}_{1}}{2L_{2}L_{3}}=\cos\theta_{2}\cos\theta_{3}+\sin\theta_{2}\sin\theta_{3}-\frac{L_{1}}{L_{3}}\cos\theta_{2}-\frac{L_{1}}{L_{2}}\cos\theta_{3}$

 

아래와 같이 치환해줍니다. 

 

$A=\cos\theta_{2}\cos\theta_{3}+\sin\theta_{2}\sin\theta_{3}-B\cos\theta_{2}-C\cos\theta_{3}$

 

아래와 같이 이항합니다. 

 

$A+B\cos\theta_{2}=\cos\theta_{2}\cos\theta_{3}+\sin\theta_{2}\sin\theta_{3}-C\cos\theta_{3}$

 

아래와 같이 묶어줍니다. 

 

$A+B\cos\theta_{2}=\left ( \cos\theta_{2}-C \right )\cos\theta_{3}+\sin\theta_{2}\sin\theta_{3}$

 

아래 성질을 이용하여 치환하겠습니다. 

 

$\sin\theta_{3}=\frac{2\tan\left ( \frac{\theta_{3}}{2} \right )}{1+\tan^{2}\left ( \frac{\theta_{3}}{2} \right )}$ 

 

$\cos\theta_{3}=\frac{1-\tan^{2}\left ( \frac{\theta_{3}}{2} \right )}{1+\tan^{2}\left ( \frac{\theta_{3}}{2} \right )}$

 

치환하면 아래와 같습니다. 편의상 $\tan^{2}\left ( \frac{\theta_{3}}{2} \right )$를 t로 놓겠습니다. 

 

$A+B\cos\theta_{2}=\left ( \cos\theta_{2}-C \right )\frac{1-t^{2}}{1+t^{2}}+\sin\theta_{2}\frac{2t}{1+t^{2}}$

 

아래와 같이 변형합니다. 

 

$\left ( A+B\cos\theta_{2} \right )\left ( 1+t^{2} \right )=\left ( \cos\theta_{2}-C \right )\left ( 1-t^{2} \right )+\sin\theta_{2}2t$

 

t에 대해 내림차순으로 정렬합니다. 

 

$\left ( A+B\cos\theta_{2}+\cos\theta_{2}-C \right )t^{2}-2\sin\theta_{2}t+A+B\cos\theta_{2}-\cos\theta_{2}+C=0$

 

아래와 같이 치환합니다. 

 

$at^{2}+bt+c=0$

 

근을 구하면 아래와 같습니다. 

 

$t=\tan\frac{\theta_{3}}{2}=\frac{-b\pm \sqrt{b^{2}-4ac}}{2a}$

 

$\theta_{3}$는 아래와 같이 계산됩니다. 

 

$\theta_{3}=2 \tan^{-1} \left ( \frac{-b\pm \sqrt{b^{2}-4ac}}{2a} \right )$

 

 

2) $\theta_{4}$ 구하기

아래 내용으로 돌아갑시다. 실수부와 허수부를 따로 나타낸 부분입니다. 

 

$L_{2}\cos\theta_{2}+L_{3}\cos\theta_{3}-L_{1}-L_{4}\cos\theta_{4}=0$

 

$L_{2}\sin\theta_{2}+L_{3}\sin\theta_{3}-L_{4}\sin\theta_{4}=0$

 

$\theta_{3}$를 제거하기 위해 위 두 식을 각각 아래와 같이 이항해줍니다.

 

$L_{3}\cos\theta_{3}=-L_{2}\cos\theta_{2}+L_{4}\cos\theta_{4}+L_{1}$

 

$L_{3}\sin\theta_{3}=-L_{2}\sin\theta_{2}+L_{4}\sin\theta_{4}$

 

위 두 식의 양변을 제곱하여 더해줍니다. 

 

$\begin{align} L_{3}^{2}\left ( \cos^{2}\theta_{3}+\sin^{2}\theta_{3} \right )=&L^{2}_{2}\cos^{2}\theta_{2}+L^{2}_{4}\cos^{3}\theta_{4}+L^{2}_{1}-2L_{2}L_{4}\cos\theta_{2}\cos\theta_{4}-2L_{1}L_{2}\cos\theta_{2}+2L_{1}L_{4}\cos\theta_{4}+ \\&L_{2}^{2}\sin^{2}\theta_{2}+L_{4}^{2}\sin^{2}\theta_{4} +2L_{2}L_{4}\sin\theta_{2}\sin\theta_{4}\end{align}$

 

$\cos^{2}\theta+\sin^{2}\theta=1$이므로 아래와 같이 변형됩니다. 

 

$L_{3}^{2}=L^{2}_{2}+L^{2}_{4}+L^{2}_{1}-2L_{2}L_{4}\cos\theta_{2}\cos\theta_{4}-2L_{2}L_{4}\sin\theta_{2}\sin\theta_{4}-2L_{1}L_{2}\cos\theta_{2}+2L_{1}L_{4}\cos\theta_{4}$

 

아래와 같이 이항합니다. 

 

$L_{3}^{2}-L^{2}_{2}-L^{2}_{4}-L^{2}_{1}=-2L_{2}L_{4}\cos\theta_{2}\cos\theta_{4}-2L_{2}L_{4}\sin\theta_{2}\sin\theta_{4}-2L_{1}L_{2}\cos\theta_{2}+2L_{1}L_{4}\cos\theta_{4}$

 

양 변을 $-2L_{2}L_{4}$로 나눠줍니다. 

 

$\frac{L_{3}^{2}-L^{2}_{2}-L^{2}_{4}-L^{2}_{1}}{-2L_{2}L_{4}}=\cos\theta_{2}\cos\theta_{4}+\sin\theta_{2}\sin\theta_{4}+\frac{L_{1}}{L_{4}}\cos\theta_{2}-\frac{L_{1}}{L_{2}}\cos\theta_{4}$

 

아래와 같이 치환합니다. 

 

$E=\cos\theta_{2}\cos\theta_{4}+\sin\theta_{2}\sin\theta_{4}+F\cos\theta_{2}-G\cos\theta_{4}$

 

아래와 같이 이항합니다. 

 

$E-F\cos\theta_{2}=\cos\theta_{2}\cos\theta_{4}+\sin\theta_{2}\sin\theta_{4}-G\cos\theta_{4}$

 

아래 성질을 이용하여 치환하겠습니다.

 

$\sin\theta_{4}=\frac{2\tan\left ( \frac{\theta_{4}}{2} \right )}{1+\tan^{2}\left ( \frac{\theta_{4}}{2} \right )}$ 

 

$\cos\theta_{4}=\frac{1-\tan^{2}\left ( \frac{\theta_{4}}{2} \right )}{1+\tan^{2}\left ( \frac{\theta_{4}}{2} \right )}$

 

치환하면 아래와 같습니다. 편의상 $\tan^{2}\left ( \frac{\theta_{4}}{2} \right )$를 t로 놓겠습니다. 

 

$E-F\cos\theta_{2}=\cos\theta_{2}\frac{1-t^{2}}{1+t^{2}}+\sin\theta_{2}\frac{2t}{1+t^{2}}-G\frac{1-t^{2}}{1+t^{2}}$

 

아래와 같이 변형합니다. 

 

$\left ( E-F\cos\theta_{2} \right )\left ( 1+t^{2} \right )=\cos\theta_{2}\left ( 1-t^{2} \right )+\sin\theta_{2}2t-G\left ( 1-t^{2} \right )$

 

t에 대해 내림차순으로 정리합니다. 

 

$\left ( E-F\cos\theta_{2}+\cos\theta_{2}-G \right )t^{2}-2\sin\theta_{2}t+E-F\cos\theta_{2}-\cos\theta_{2}+G=0$

 

아래와 같이 치환합니다. 

 

$et^{2}+ft+g=0$

 

$t=\tan\frac{\theta_{4}}{2}=\frac{-f\pm \sqrt{f^{2}-4eg}}{2e}$

 

$\theta_{4}$는 아래와 같이 계산됩니다. 

 

$\theta_{4}=2 \tan^{-1} \left ( \frac{-f\pm \sqrt{f^{2}-4eg}}{2e} \right )$

---

아래는 $\theta_{3}$ 계산 요약입니다. 

 

$\theta_{3}=2 \tan^{-1} \left ( \frac{-b\pm \sqrt{b^{2}-4ac}}{2a} \right )$

 

 

$a=A+B\cos\theta_{2}+\cos\theta_{2}-C$

 

$b=-2\sin\theta_{2}$

 

$c=A+B\cos\theta_{2}-\cos\theta_{2}+C$

 

 

$A=\frac{L_{4}^{2}-L^{2}_{2}-L^{2}_{3}-L^{2}_{1}}{2L_{2}L_{3}}$

 

$B=\frac{L_{1}}{L_{3}}$

 

$C=\frac{L_{1}}{L_{2}}$

 

---

아래는 $\theta_{4}$ 계산 요약입니다.

 

$\theta_{4}=2 \tan^{-1} \left ( \frac{-f\pm \sqrt{f^{2}-4eg}}{2e} \right )$

 

 

$e=E-F\cos\theta_{2}+\cos\theta_{2}-G$

 

$f=-2\sin\theta_{2}$

 

$g=E-F\cos\theta_{2}-\cos\theta_{2}+G$

 

 

$E=\frac{L_{3}^{2}-L^{2}_{2}-L^{2}_{4}-L^{2}_{1}}{-2L_{2}L_{4}}$

 

$F=\frac{L_{1}}{L_{4}}$

 

$G=\frac{L_{1}}{L_{2}}$